6 6 Systems Of Inequalities

Slides: 18

Download presentation

6 -6 Solving Systems of Linear Inequalities Objective Graph and solve systems of linear

6 -vi Solving Systems of Linear Inequalities Objective Graph and solve systems of linear inequalities in two variables. Vocabulary system of linear inequalities solution of a system of linear inequalities Holt Algebra ane

6 -six Solving Systems of Linear Inequalities i. Graph y<x+2 5 x + 2

six -6 Solving Systems of Linear Inequalities one. Graph y<x+2 v 10 + ii y ≥ 10 2. Graph y > 3 10 – 2 y < 3 ten + vi Give 2 ordered pairs that are solutions and ii that are not solutions. three. Dee has at near $150 to spend on restocking dolls and trains at her toy shop. Dolls price $7. 50 and trains price $5. 00. Dee needs no more than ten trains and she needs at least eight dolls. Prove and depict all possible combinations of dolls and trains that Dee can buy. List two possible combinations. Holt Algebra 1

6 -6 Solving Systems of Linear Inequalities Example 1: Identifying Solutions of Systems of

6 -six Solving Systems of Linear Inequalities Example one: Identifying Solutions of Systems of Linear Inequalities Tell whether the ordered pair is a solution of the given system. (– i, 5); y < – 2 x – 1 y≥10+3 (– 1, 5) y < – ii x – 1 5 – 2(– one) – 1 5 2– 1 5 < ane (– one, 5) y≥ten+3 5 – 1 + 3 v ≥ 2 (– i, 5) is non a solution to the organization because it does not satisfy both inequalities. Holt Algebra 1

6 -6 Solving Systems of Linear Inequalities Remember! An ordered pair must be a

half dozen -6 Solving Systems of Linear Inequalities Remember! An ordered pair must be a solution of all inequalities to be a solution of the system. Holt Algebra 1

6 -6 Solving Systems of Linear Inequalities A system of linear inequalities is a

half dozen -6 Solving Systems of Linear Inequalities A system of linear inequalities is a set up of 2 or more linear inequalities containing ii or more variables. The solutions of a organisation of linear inequalities consists of all the ordered pairs that satisfy all the linear inequalities in the arrangement. To show all the solutions of a system of linear inequalities, graph the solutions of each inequality. The solutions of the organisation are represented past the overlapping shaded regions. To a higher place are graphs of Examples 1 A and 1 B on p. 421. Holt Algebra one

6 -6 Solving Systems of Linear Inequalities Example 2 A: Solving a System of

6 -6 Solving Systems of Linear Inequalities Example 2 A: Solving a Organization of Linear Inequalities by Graphing Graph the system of linear inequalities. Give 2 ordered pairs that are solutions and two that are not solutions. y≤ 3 y > –x + 5 (– 1, 4) Graph the organization. y≤ iii y > –ten + v (8, i) and (six, 3) are solutions. (– 1, four) and (2, 6) are not solutions. Holt Algebra 1 (2, six) (6, 3) (viii, i)

6 -6 Solving Systems of Linear Inequalities Example 2 B: Solving a System of

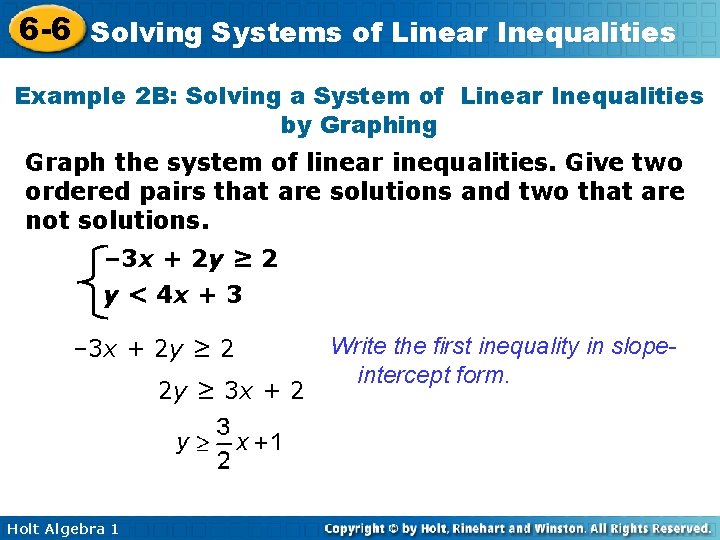
half dozen -half-dozen Solving Systems of Linear Inequalities Example 2 B: Solving a Organization of Linear Inequalities past Graphing Graph the system of linear inequalities. Give two ordered pairs that are solutions and 2 that are not solutions. – 3 x + 2 y ≥ 2 y < iv x + three – 3 10 + 2 y ≥ ii 2 y ≥ three ten + 2 Holt Algebra 1 Write the first inequality in slopeintercept form.

6 -6 Solving Systems of Linear Inequalities Example 2 B Continued Graph the system.

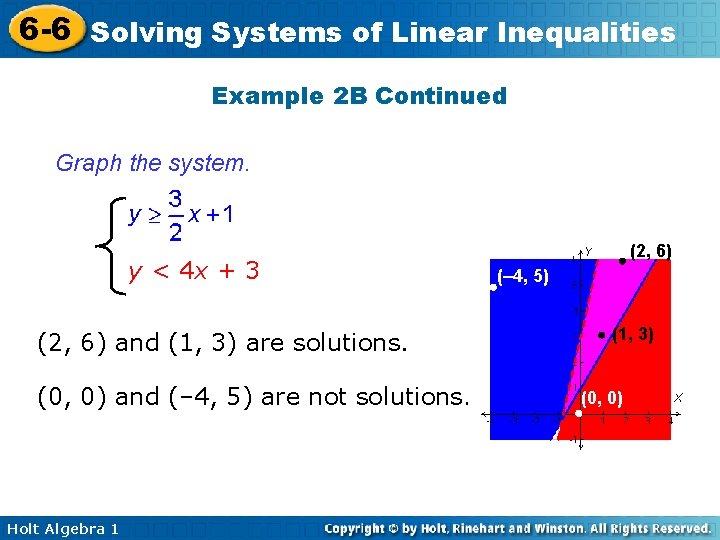
6 -6 Solving Systems of Linear Inequalities Example 2 B Continued Graph the organisation. y < 4 ten + iii (2, 6) and (1, 3) are solutions. (0, 0) and (– 4, 5) are not solutions. Holt Algebra 1 (– 4, 5) (two, 6) (1, 3) (0, 0)

6 -6 Solving Systems of Linear Inequalities Example 2 C Graph the system of

6 -six Solving Systems of Linear Inequalities Example 2 C Graph the system of linear inequalities. Give 2 ordered pairs that are solutions and two that are not solutions. y>x– 7 three x + half-dozen y ≤ 12 Write the second inequality in 6 y ≤ – 3 x + 12 slope-intercept course. y≤ Holt Algebra one ten+ii

6 -6 Solving Systems of Linear Inequalities Example 2 C Continued Graph the system.

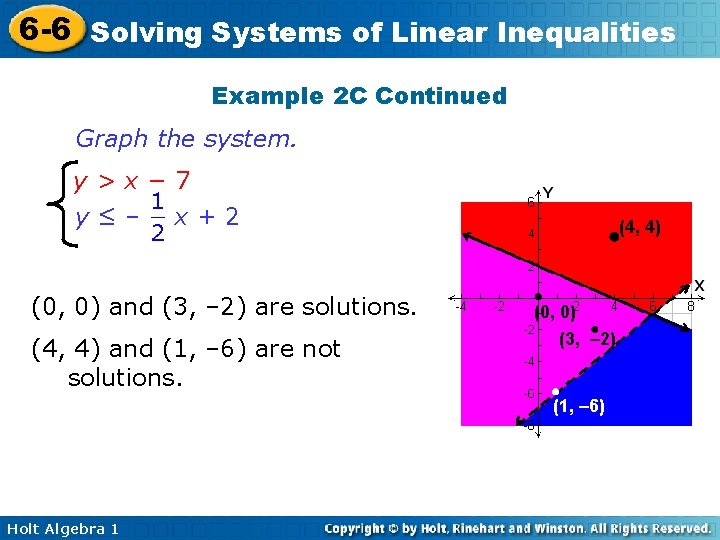
6 -6 Solving Systems of Linear Inequalities Example ii C Continued Graph the system. y>x− 7 y≤– x+2 (0, 0) and (three, – 2) are solutions. (4, 4) and (1, – 6) are not solutions. Holt Algebra 1 (iv, 4) (0, 0) (3, – 2) (one, – 6)

6 -6 Solving Systems of Linear Inequalities Example 3 At her party, Alice is

6 -six Solving Systems of Linear Inequalities Example 3 At her party, Alice is serving pepper jack cheese and cheddar cheese. She wants to have at least 2 pounds of each. Alice wants to spend at nigh $20 on cheese. Show and describe all possible combinations of the two cheeses Alice could buy. Listing two possible combinations. Price per Pound ($) Holt Algebra i Pepper Jack 4 Cheddar two

6 -6 Solving Systems of Linear Inequalities Example 3 Continued Step 1 Write a

6 -half dozen Solving Systems of Linear Inequalities Example 3 Continued Pace 1 Write a organisation of inequalities. Let x represent the pounds of cheddar and y represent the pounds of pepper jack. 10≥ 2 y≥ 2 2 10 + iv y ≤ 20 Holt Algebra 1 She wants at least 2 pounds of cheddar. She wants at to the lowest degree two pounds of pepper jack. She wants to spend no more than than $20.

6 -6 Solving Systems of Linear Inequalities Example 3 Continued Step 2 Graph the

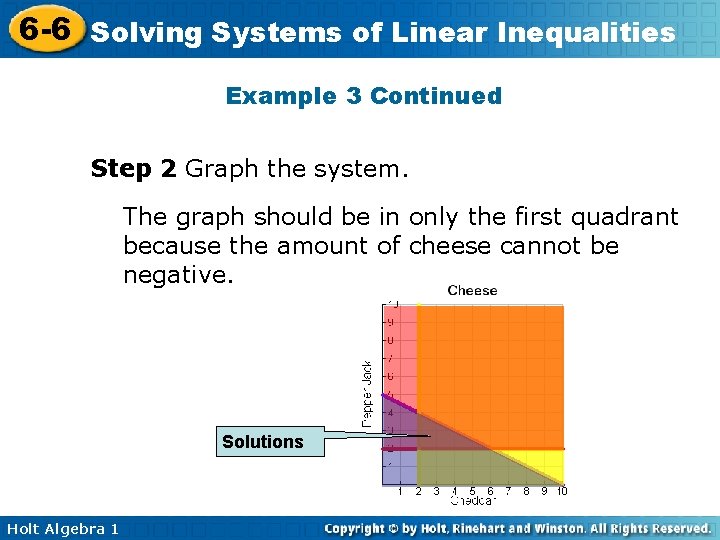
6 -6 Solving Systems of Linear Inequalities Example 3 Continued Step 2 Graph the system. The graph should exist in only the first quadrant because the amount of cheese cannot exist negative. Solutions Holt Algebra 1

6 -6 Solving Systems of Linear Inequalities Step 3 Describe all possible combinations. All

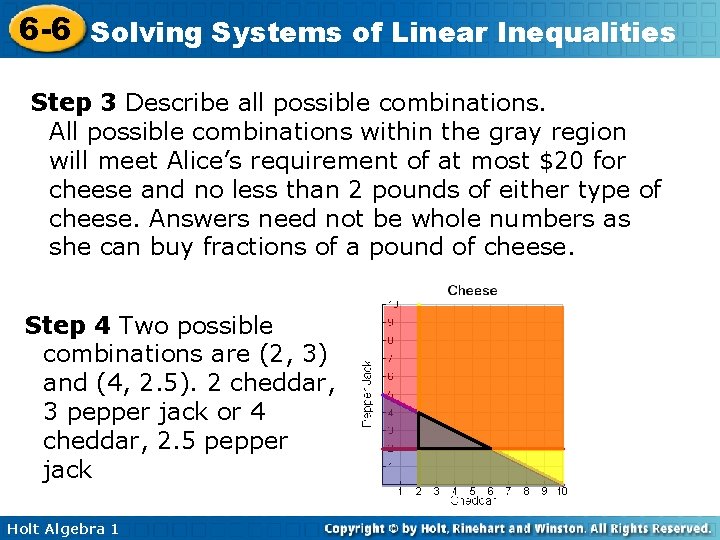
6 -half dozen Solving Systems of Linear Inequalities Pace iii Draw all possible combinations. All possible combinations within the grey region volition see Alice'south requirement of at nigh $xx for cheese and no less than 2 pounds of either type of cheese. Answers need not be whole numbers as she can purchase fractions of a pound of cheese. Step four Two possible combinations are (2, iii) and (4, 2. 5). 2 cheddar, 3 pepper jack or 4 cheddar, 2. v pepper jack Holt Algebra 1

half-dozen -six Solving Systems of Linear Inequalities Notes one. Graph y<x+2 5 x +

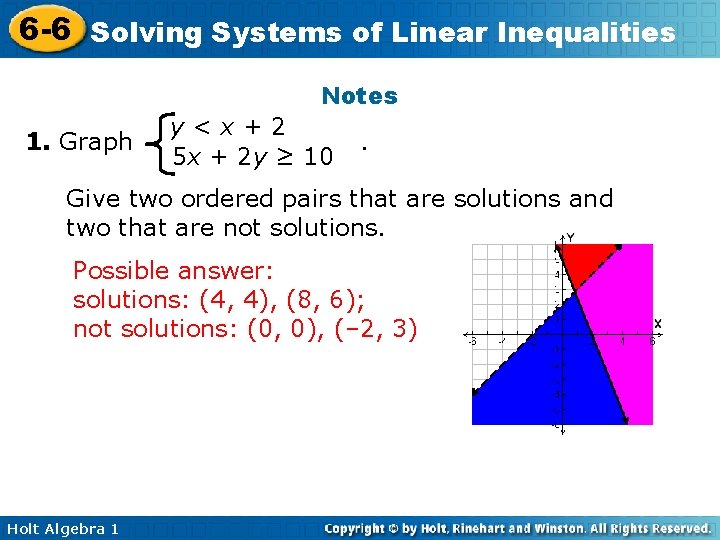
half-dozen -6 Solving Systems of Linear Inequalities Notes 1. Graph y<x+2 5 x + 2 y ≥ 10 . Requite ii ordered pairs that are solutions and two that are non solutions. Possible respond: solutions: (4, 4), (8, 6); not solutions: (0, 0), (– ii, 3) Holt Algebra one

6 -6 Solving Systems of Linear Inequalities Notes 2. Graph the system of linear

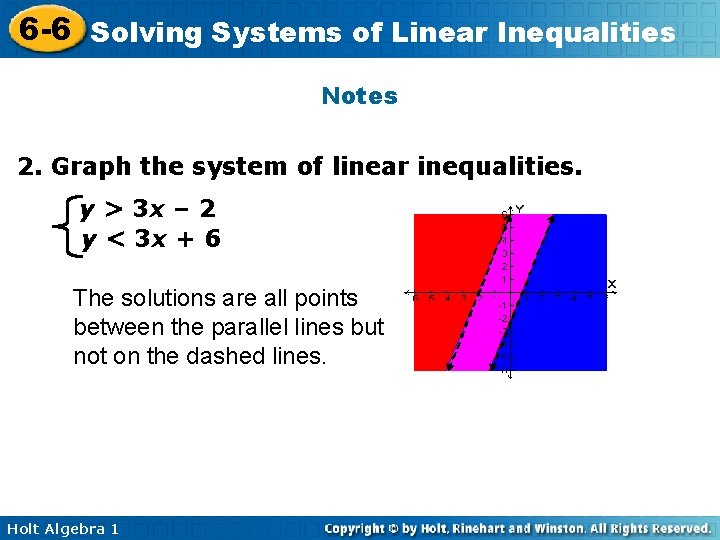
six -6 Solving Systems of Linear Inequalities Notes ii. Graph the system of linear inequalities. y > 3 10 – 2 y < 3 10 + six The solutions are all points betwixt the parallel lines only non on the dashed lines. Holt Algebra one

6 -6 Solving Systems of Linear Inequalities Notes 3. Dee has at most $150

6 -6 Solving Systems of Linear Inequalities Notes three. Dee has at almost $150 to spend on restocking dolls and trains at her toy store. Dolls cost $7. l and trains price $5. 00. Dee needs no more than than ten trains and she needs at least viii dolls. Show and describe all possible combinations of dolls and trains that Dee can buy. List two possible combinations. Holt Algebra 1

6 -6 Solving Systems of Linear Inequalities Notes #3: Continued Reasonable answers must be

6 -half dozen Solving Systems of Linear Inequalities Notes #3: Continued Reasonable answers must be whole numbers. Possible answer: (12 dolls, 6 trains) and (16 dolls, 4 trains) Solutions Holt Algebra 1